How Nature Does its Sums |
If DNA is the building blocks of biology then maths maybe the builder,finds
Simon Singh
It is very rare that I find myself standing in my local supermarket staring at the surface of a pineapple.But that is what happened soon after I heard Ian Stewart give a lecture on the subject of his latest book. Stewart,who is one of Britain's most prominent mathematicians,has recently become interested in a new area of research at the frontier between biology and mathematics,which is already providing some startling insights into the living world......including pineapples.

Biomathematics is a reaction against the worship of DNA,and the opinion that
genes govern all aspects of of life [Genetic Determinism
-LB]. DNA is essentially a complex set of instructions,and in recent
decades there has been a growing belief that it is this strand of molecules
which guides all aspects of life.
|
|
|
| A cross section through bamboo showing the arrangement of fibre bundles | Pine cones with two opposing spirals - nature exploiting maths |
The seed head of a flower - such arrangements maximise the packing of seeds |
However,if one looks at the instructions that would be required to dictate
the nerve by nerve construction of the human nervous system, then it is clear
that our DNA cannot carry enough information to complete this task,let alone
all the others. Biomathematics claims that the information gap can be closed
by invoking mathematics and the laws of physics.
Physics and mathematics are capable of producing intricate patterns in
non-organic constructions (for example,
snowflakes
and sand dunes). They can offer a range of patterns
which will emerge
spontaneously, given the correct starting conditions.The theory which
is currently gaining support says that life operates by using DNA to create
the right starting conditions,and thereafter physics and maths do all the
rest,DNA is not the secret of life - hence the title of the book
(Life's Other Secret: The New Mathematics of the Living World -
Ian Stewart).
The strange case of the fascinating pineapple begins with the observation
that its surface is covered in diamonds,which form two sets of spirals,twisting
in opposite directions.There are 8 sloping to the left,and 13 sloping to
the right.These numbers are special because they are part of
the Fibonacci
sequence (1,1,2,3,5,8,13,21,34,55...),in which the next number
in the series is generated by adding the two previous numbers,ie 1+1=2 and
1+2=3,and so on.
 |
Pattern by Numbers Seeds in a sunflower head are usually arranged in 34 clockwise and 55 anti- clockwise spirals |
The sequence crops up in many other plants,such as in the arrangement
of seeds in the head of a sunflower which usually has 34 spirals going
clockwise,and 55 going anti-clockwise.So far,this is just an observation,but
biomathematics seeks to find an explanation.
An elegant argument in Ian Stewart's book explains that evolution encourages
as many seeds as possible,physics provides the mechanism for packing them
in most tightly,and mathematics leads to the Fibonacci sequence.
Stewart describes beautifully a whole series of of biological phenomena which
can be elucidated by mathematics: the formation of the stomach in a frog
embryo,the life cycle of the slime mould [Ref:Prof Bonner - Slime
Moulds Cyclic AMP],the patterns on
the skin of angel fish and tigers,the movement of bipeds,quadrupeds and
hexapods,and animal behaviour.He does not have all the answers,but bear in
mind that biomathematics is a recent development in science,and as compensation
we get to see a subject full of controversy and youthful enthusiasm.

Finally,there may be people who suspect that the influence of mathematics can only serve to provide a more sterile view of life.This is not the case.The combination of DNA and mathematics enriches biology,and magnifies the wonder of life.Personally,I have found that my love of pineapples has grown enormously since learning about the mathematics of its surface.
 |
Simon Singh is a television producer and author of "Fermat's Last Theorem" (Fourth Estate).
|
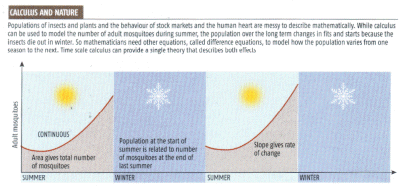
![The erratic stop-start of the real world has long defeated mathematicians,but now they have a new trick up their sleeves. It's called time scale calculus,and everything from mosquitos to market traders will bow before it. Vanessa Spedding reports.[New Scientist 19Jul,2003]](graphics/natsums/MESSY4.gif) IS
THE world a hopelessly untidy place? It all depends on your point of view
of course. Biologists might see it as full of the rich diversity of nature.
Physicists, perhaps view the world as containing an infinite array of phenomena
to explore. To mathematicians, however, it is just a mess. For instance,
they have struggled to use maths to describe something as apparently simple
as the way insect populations vary from one season
to the next. The problem is that one mathematical theorem describes how the
adult population changes from day to day, while a totally different approach
is needed to explain what happens over the long term, where numbers can change
dramatically over different seasons. And you can't just bring these two bits
of maths together to get an accurate picture. IS
THE world a hopelessly untidy place? It all depends on your point of view
of course. Biologists might see it as full of the rich diversity of nature.
Physicists, perhaps view the world as containing an infinite array of phenomena
to explore. To mathematicians, however, it is just a mess. For instance,
they have struggled to use maths to describe something as apparently simple
as the way insect populations vary from one season
to the next. The problem is that one mathematical theorem describes how the
adult population changes from day to day, while a totally different approach
is needed to explain what happens over the long term, where numbers can change
dramatically over different seasons. And you can't just bring these two bits
of maths together to get an accurate picture.
The problem plagues more than just studies of insect populations. Many phenomena,
from combustion and stock markets to epidemics
and crop harvests, present the same difficulty. Researchers simply have no
way of predicting the behaviour of things that change smoothly at one level,
but fitfully at another. But that might be about to change. Take the work of mathematician Diana Thomas at Montclair State University in New Jersey. Thomas models populations of mosquitoes that carry West Nile virus, and time scale calculus, she believes, is the answer to all her problems. Suppose you count up the number of mosquitoes in a city every day. During the summer months, a steady stream of mosquito larvae mature into adults and then spend the next few weeks dodging predators such as birds and bats, and people trying to swat them. Plot the mosquito population against time through the summer and you could draw a smooth curve through the data without lifting your pencil off the page. Mathematicians call this continuous behaviour, and can almost always write down a mathematical formula to describe the shape of the curve. Such an equation is incredibly powerful. If you want to forecast the number of mosquitoes on any given day, for instance, you just plug the date into the formula and out pops the answer. And you can work out much more by calling on the theory of calculus devised by Newton and Leibniz. Suppose you want to know how many mosquitoes had infested the city so far that year. Calculus says you simply calculate the area under the curve. Meanwhile the slope of the curve tells you how fast the population is growing or shrinking from one moment to the next. That's all fine while life runs smoothly during the summer. But it is a different story for the rest of the year. In winter the adult mosquitoes die off completely so the curve drops to zero. The eggs lie dormant until the spring, when a new generation of mosquitoes emerges and the population rises again. So if you were plotting several years' worth of data, you would see a series of disconnected curves (see below).
Researchers have developed a separate type of mathematics, called difference
calculus, to deal with this "discrete", stop-start behaviour. Difference
calculus gives you the equations to describe jumps. Difference equations are not only useful in situations with discrete time jumps. When you have a messy problem to solve, it is often much easier to approximate it using difference equations rather than write down an exact continuous equation. And that's exactly what Thomas did in her work on mosquitoes carrying West Nile virus. Four years ago she started using difference equations to model the number of such mozzies in New York City. The virus, which lives mainly in birds, can be transmitted to humans, where it can cause a range of flu-like symptoms and, at worst, kill. Since West Nile arrived in New York in 1999 it has spread westwards in waves across the whole of North America. In an effort to control the mosquitoes in New York, public health officials spray insecticide throughout the city each summer. But so far, these efforts have largely failed.
Tidy sums
The problem was that Thomas's model was just too simplistic. Although her
equations could model certain aspects of the virus, they failed with others.
West Nile doesn't stay strictly dormant in winter, as Thomas's difference
equations assumed. As winter approaches and the mosquitoes in New York die,
migrating birds carry the virus to warmer southern states, as well as Mexico
and the Caribbean. Local mosquitoes catch West Nile from infected migrant
birds and pass it on to healthy ones. So the virus spreads continually throughout
the year, as more birds fall victim to it.
Unknown to her, a young mathematician had already done just that. In 1988,
as a graduate student at the University of Augsburg in Germany, Stefan Hilger
worked on some of the mathematical tools for modelling change. His adviser
Bernd Aulbach asked Hilger if he could rewrite a theorem that worked for
continuous variables so that it worked for discrete inputs too. When he looked
closely at the two theorems, he found they weren't so different after all.
He found that when he plugged integers (...-2, -1,0.1.2..) -into his new theory the equations of difference calculus emerged the gaps betiveen the integers bring out discrete or stop-start behaviour from the theory. And when he fed in the full continuum of real numbers - integers and all the numbers in between. such as fractions and numbers like Ö2 - the theory took on the form of standard calculus. Since the set of real numbers is continuous, it brings out the continuous characteristics of calculus.
Hilger had formulated nothing less than a grand unified theory for calculus.
He called it time scale calculus - to mathematicians, "time scales" are simply
different kinds of numbers: integers, or real numbers, for example. But while
Hilger's colleagaes were impressed by the neatness of his theory, they couldn't
see any practical use for it. So when his funding dried up 3 years later,
time scale calculus seemed doomed to become a mathematical curio. The breakthrough was to realise that the time scale approach not only unites calculus and difference equations, it could also solve other problems that were a mix of stop-start and continuous behaviour. Over the next 5 years he threw himself into his research, reformulating many of the mathematical tricks of the trade so that they worked for time scale calculus. Mathematicians started to take notice of time scale calculus soon after Bohner wrote a book about it with Al Peterson at the University of Nebraska in Lincoln.
Equations for the real world
Though the latest West Nile model is not yet fully developed, Thomas and
Hartman believe that once it is complete it will provide real ammunition
in the battle against the disease. By providing accurate forecasts of where
future virus hot spots are likely to emerge, the pair hope the model will
lead to more effective health-policy decisions.
Hoffacker urges caution about premature judgements on the merits of time
scale calculus, and admits, "We don't have an application that is finished
yet:" However, she is pushing it further, and has teamed up with Billur
Kaymakçalan, a mathematician at Georgia Southern University in Statesboro,
to see if time scale theory could model electrical
activity in the heart. You only have to look at a trace from an
electrocardiogram to see that every heartbeat involves a huge spike in electrical
activity followed by a period of calm.
Sheng's work shows that time scale calculus can be useful where the sudden
changes in behaviour are spatial as well as temporal. Indeed, he predicts
that anything where there is a boundary between large and small-scale behaviour,
such as the interfaces of nanoscale structures embedded in other materials,
will benefit from the new unified approach. "It will merge into mainstream
scientific computation sooner or later," he predicts.
Vanessa Spedding is a science writer based in the UK. Additional
reporting by Valerie Jamieson |
| UNIVERSAL VEINS. Despite the stunning variety of leaf shapes
from one plant variety to another, a universal formula may guide the vein
patterns in all leaves. Researchers at the Laboratorie de Physique Statistique
(S. Bohn, 33-1-4432-3447,
[email protected]) and the Museum National
d'Historie Naturalle, both in Paris, recently studied the networks of veins
in leaves from several plant types. An analysis of leaf vein networks revealed
simple relationships between the angles that veins form when they intersect
and the thickness of the veins at the intersections. At a three-way junction
of similarly sized veins, the angles between the veins are all roughly 120
degrees (see image at
www.aip.org/mgr/png). At locations
where a small vein joins a large vein, however, the angles between the small
vein and the larger one approach 90 degrees. Imagine, for example, the angles
formed by pulling on a light thread tied to a taut rope. The similarity between
a network of threads and ropes exerting forces on each other and the patterns
in leaf veins suggested to the researchers that there is an underlying mechanism
in leaf formation that is reminiscent of simple mechanics problems. The model
breaks with theories of leaf networks related to soap froths (which require
all intersection angles to equal 120 degrees), crack propagation (with 90
degree intersections where new cracks meet old cracks), and
Turing diffusion
(which leads to patterns that resemble spreading tree branches, rather
than the net-like patterns found in leaf veins). Some botanists had considered
leaf veins as potential tools for categorizing plants. The universal similarity
of vein structure from one plant to another suggests that the patterns provide
insight to leaf mechanics, but are of little help in distinguishing or cataloging
plants. (S. Bohn et al., Phys. Rev. E, June 2002; text at
www.aip.org/physnews/select) |
"There is not a fine tree producing rotten fruit;again there
is not a rotten tree producing fine fruit. For each tree is known by
its own fruit"
- Luke 6:43,44 -quoted in "What has religion done for mankind?"
| "Even from the standpoint of the skeptic,a reasonable and
candid search into the unknown,by the light of what is known,will guide the
ubiased,intelligent reasoner in the direction of truth. Yet it is evident
that without a direct revelation of the plans and
purposes of God,men could only approximate the
truth,and arrive at indefinite conclusions.But let us for the moment lay
aside the Bible,and look at things from the standpoint of reason
alone. He who can look into the sky with a telescope,or even with his natural eye alone,and see its symmetry,beauty,order,harmony and diversity,and yet doubt the Creator of these is vastly his superior both in wisdom and power,or who can suppose for a moment that such order came by chance,without a creator,has so far lost or ignored the faculty of reason as to be properly considered what the Bible terms him,a fool (one who ignores or lacks reason):"The fool hath said in his heart,There is no God." However it happened,at least that much of the Bible is true,as every reasonable mind must conclude;for it is a self evident truth that effects must be produced by competent causes.Every plant and every flower,even speaks volumes of testimony on this subject. Intricate in construction,exquisitely beautiful in form an texture,each speaks of wisdom and skill above human.How short sighted the absurdity which boasts of human skill and ingenuity,and attributes to mere chance the regularity,uniformity and harmony of nature;which acknowledges the laws of nature,while denying that nature has an intelligent Lawgiver." -"The Divine Plan of the Ages" [Ignorant nonsense-LB] |
Life's Other Secret: The New Mathematics of the Living
World - Ian Stewart |






 Further Reading
Further Reading